Scattering geometry

The scattering geometry in Nexus is defined with respect to the photon coordinate system

\[\begin{split}\begin{bmatrix} \sigma \\ \pi \\ k \end{bmatrix}\end{split}\]

where \(\sigma\) and \(\pi\) are the two independent linear polarization axes of the photon and \(k\) is the k-vector of the photon.

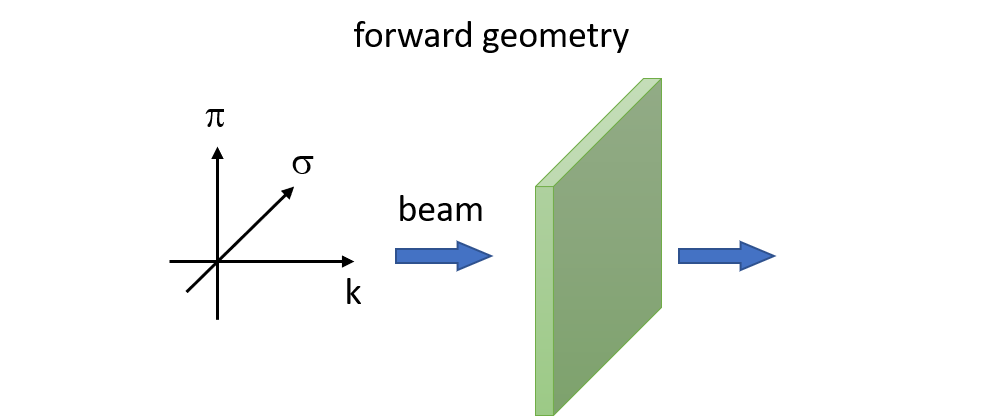
Forward scattering

A forward scattering geometry corresponds to an extended sample in the plane of the polarization axes.

Grazing incidence scattering

A grazing-incidence scattering geometry corresponds to an extended sample (at an incidence angle of 0 degree) in the plane spanned by \(k\) and an arbitrary axis in the polarization plane. The direction of the second axis in the polarization plane is not important as gracing incidence scattering is formally treated like forward scattering in the implemented theory. The formalism is therefore only valid at small incidence angles. The typical scattering geometry in an experiment is shown here

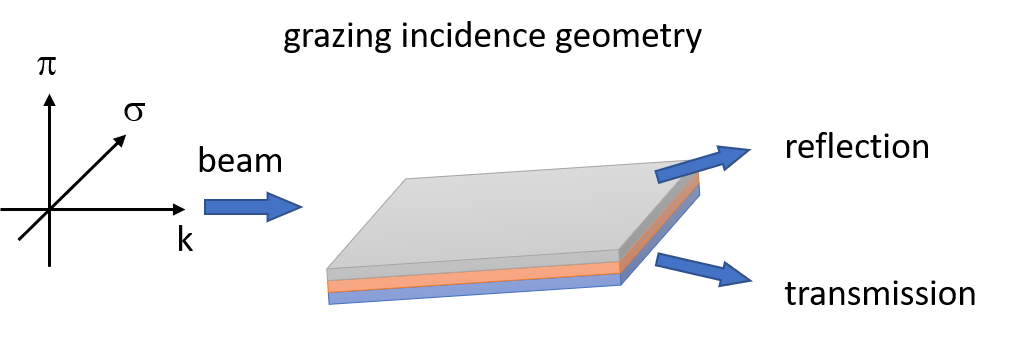
One could also rotate the sample around \(k\) by any angle.

Hyperfine Interactions

The hyperfine fields are also defined with respect to the photon system.

Magnetic hyperfine field

For the magnetic field its field strength and two angles are given. The polar angle \(\vartheta\) is defined from the \(k\) vector. The azimuthal angle \(\varphi\) is defined in the \(\sigma - \pi\) plane from the \(\sigma\) direction.

Electric field gradient

The electric field gradient tensor is defined by the largest principal tensor component \(V_{zz}\), the asymmetry parameter \(\eta\), and three Euler angles. In Nexus, instead of \(V_{zz}\) the quadrupole splitting \(QS\) in mm/s is given. The angles are the extrinsic Euler angles in ZYZ convention. The angles are thus defined as rotations around the internal fixed-frame coordinate system of Nexus.